Very simple terms: A confidence interval is a statistical device for saying, "I am pretty sure the true value of a number I am approximating is within this range. How sure? I am n% sure." Where n is usually 95 or 99 and "this range" is some range of numeric values.
For example, if I ask you to estimate the number of jelly beans in a 3 gallon Jar you might reasonably say I am 90% sure the actual number is between 3,000 and 4,000 jelly beans. Without knowing it, you've just built a 90% confidence interval.
More accurate definition: When sampling from a population to estimate a mean a confidence interval is a range of values within which you are n% confident the true mean is included. n = some stated percentage, called a confidence level. If n = 95 then one can say, "In 95 out of 100 samples my estimated mean will fall within this stated range. Therefore, the true mean has a 95% chance of falling within this range. Conversely, there is a 5% chance that the true mean is not within this interval."
And we often use the alpha to denote the siginificant levle and 1-alpha to be confident level!
The p-value is defined as the probability, under the assumption of hypothesis
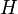H
, of obtaining a result equal to or more extreme than what was actually observed. Depending on how we look at it, the "more extreme than what was actually observed" can either mean
{ X \geq x }
(right tail event) or
{ X \leq x }
(left tail event) or the "smaller" of
{ X \leq x}
and
{ X \geq x }
(double tailed event). Thus the p-value is given by
网友评论